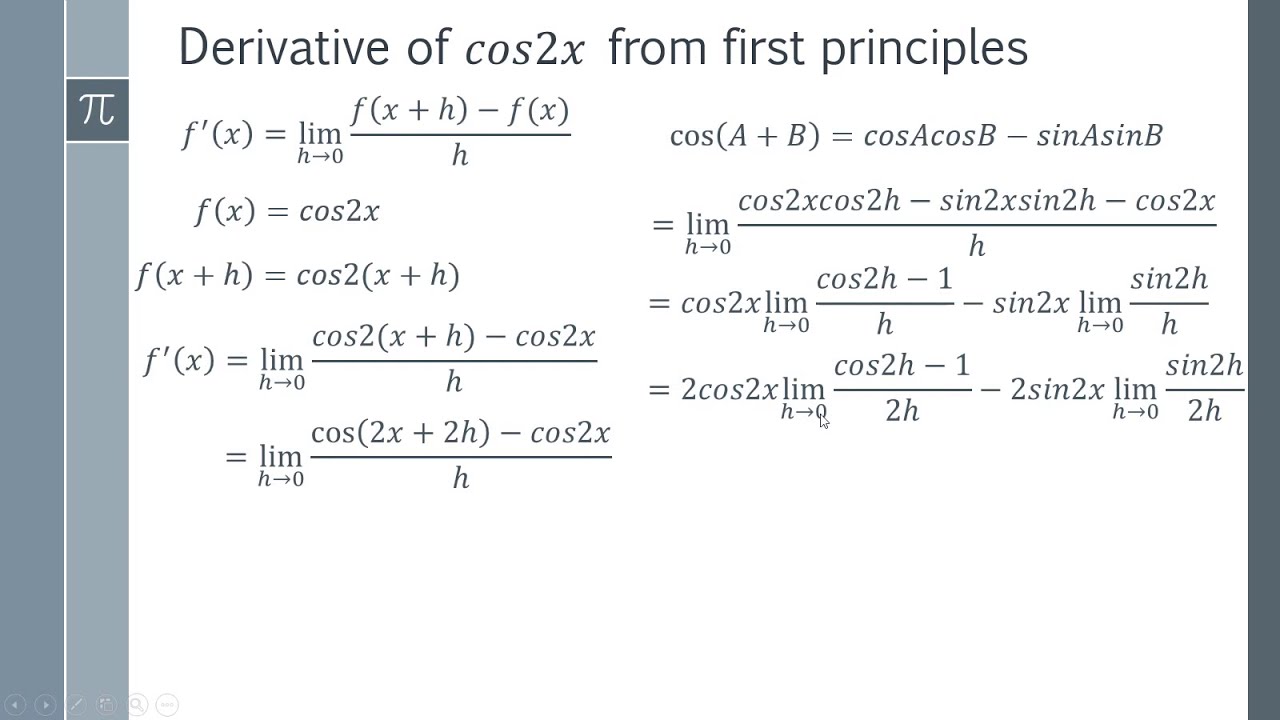The derivative of cos2x is an essential concept in calculus that plays a significant role in various applications in mathematics and science. Whether you are a student trying to master the fundamentals or a professional looking to brush up on your skills, grasping the derivative of this trigonometric function can be invaluable. The derivative of cos2x helps in understanding the behavior of the cosine function as it applies to different angles, providing insight into its rate of change, slope, and maximum and minimum values.
In this article, we will delve into the intricacies of the derivative of cos2x, exploring both its theoretical underpinnings and practical applications. We will also address common questions that arise when dealing with derivatives, especially for trigonometric functions. By the end of this guide, you will have a thorough understanding of how to compute the derivative of cos2x and how it can be applied in various mathematical scenarios.
As we navigate through the content, we will break down complex concepts into manageable sections, making it easier for anyone to follow along. Whether you’re preparing for an exam, tutoring a peer, or just curious about calculus, this article will serve as a useful resource for mastering the derivative of cos2x.
What is the Derivative of cos2x?
The derivative of cos2x refers to how fast the cosine function changes with respect to the angle 2x. To find this derivative, we utilize the chain rule, which is a fundamental principle in calculus that allows us to differentiate composite functions. The function cos2x is a composite function where the inner function is 2x and the outer function is cosine.
How to Calculate the Derivative of cos2x?
To calculate the derivative of cos2x, we apply the chain rule as follows:
- Identify the outer function: In this case, the outer function is cos(u), where u = 2x.
- Identify the inner function: The inner function is u = 2x.
- Differentiate the outer function: The derivative of cos(u) is -sin(u).
- Differentiate the inner function: The derivative of u = 2x is 2.
- Apply the chain rule: Multiply the derivative of the outer function by the derivative of the inner function.
Hence, the derivative of cos2x is:
Derivative of cos2x = -sin(2x) * (2) = -2sin(2x)
What Does the Result Mean in Practical Terms?
The result -2sin(2x) indicates the rate of change of the cosine function with respect to the angle 2x. This means that as the value of 2x changes, the slope or rate of change of the cosine function can be expressed using this derivative. This information is crucial in many fields such as physics, engineering, and economics, where understanding the behavior of waveforms and oscillations is essential.
Why is the Derivative of cos2x Important?
The derivative of cos2x has numerous applications in various domains:
- Physics: Understanding the motion of oscillating systems, such as pendulums or springs.
- Engineering: Designing systems that involve periodic functions and waveforms.
- Economics: Analyzing cyclical trends and behaviors in economic data.
- Computer Graphics: Implementing animations or simulations that involve periodic motion.
How Does the Derivative of cos2x Appear in Graphs?
When graphed, the derivative of cos2x, which is -2sin(2x), provides valuable information about the original function cos2x. The points where the derivative equals zero indicate local maxima and minima of the cosine function. This means that by analyzing the derivative, one can determine the turning points of the graph of cos2x.
What About Higher Derivatives of cos2x?
Higher derivatives can also be computed to provide more information about the behavior of the function. The second derivative of cos2x can help determine concavity and points of inflection. To find the second derivative, we differentiate the first derivative -2sin(2x):
The second derivative is:
Derivative of -2sin(2x) = -2 * 2cos(2x) = -4cos(2x)
Common Misconceptions About Derivatives of Trigonometric Functions
When learning about derivatives, especially for trigonometric functions, there are several common misconceptions that students may have:
- Assuming linearity: Derivatives are not always linear, especially for composite functions.
- Misunderstanding the chain rule: The chain rule is essential when differentiating composite functions like cos2x.
- Ignoring negative signs: The negative sign in the derivative indicates the direction of change and should not be overlooked.
How Can I Practice Finding the Derivative of cos2x?
Practice is key to mastering derivatives. Here are some effective ways to practice:
- Work through practice problems in calculus textbooks.
- Use online resources and interactive calculators to verify your answers.
- Join study groups to discuss and solve problems collaboratively.
- Teach the concept to someone else to solidify your understanding.
Are There Any Real-World Applications of the Derivative of cos2x?
Yes, the derivative of cos2x has several real-world applications, including:
- Modeling sound waves and vibrations in acoustics.
- Studying the behavior of alternating current in electrical engineering.
- Describing seasonal variations in economics and environmental studies.
Conclusion: Mastering the Derivative of cos2x
In conclusion, understanding the derivative of cos2x is fundamental for anyone studying calculus and its applications. By applying the chain rule and practicing regularly, you can become proficient in calculating derivatives for trigonometric functions. The insights gained from these derivatives not only enhance your mathematical skills but also open doors to various applications in science, engineering, and beyond. Embrace the challenge, practice diligently, and you'll find that mastering the derivative of cos2x is an achievable goal!
You Might Also Like
Unraveling The Fiery Legacy Of Firestorm In DC ComicsUnderstanding The Apple Shaped Body: A Comprehensive Guide
No SIM Available: Understanding The Frustration And Solutions
Lord Ned Stark: The Epitome Of Honor And Loyalty
Exploring The Journey Of Liam Gallagher In Shameless Season
Article Recommendations
- Justin Fields Wife
- Meghan Markle Kids
- Is Jenna Fischer Married
- Dylan Macdonald
- Mma Fight Share
- Damon Wayans
- Is Kendall Jenner Pregnant
- Randy Adams Face Accident
- Alexis Stewart Net Worth
- Is Mark Levin Well